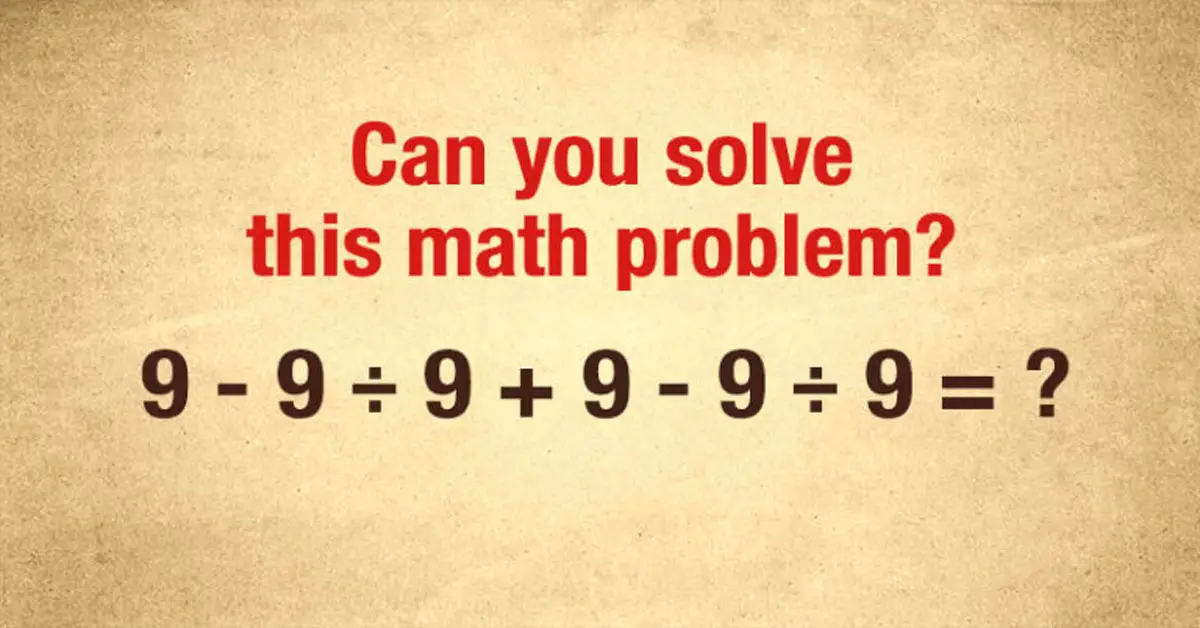A math question from the ‘50s is causing a major stir online, and most people aren’t actually able to solve it correctly without using our saving grace – the calculator. I know you’re thinking this is another one of those annoyingly difficult questions that pop up occasionally and everyone always seems to have a different answer. On the contrary, it’s probably something you encountered back at school. If you’re like me and you were not exactly one of the best math students back in middle school, this might be another chance to prove yourself. I tried it, and it’s actually one of the hardest math problems I’ve come across. Can you solve it?
The hardest math problem is this:
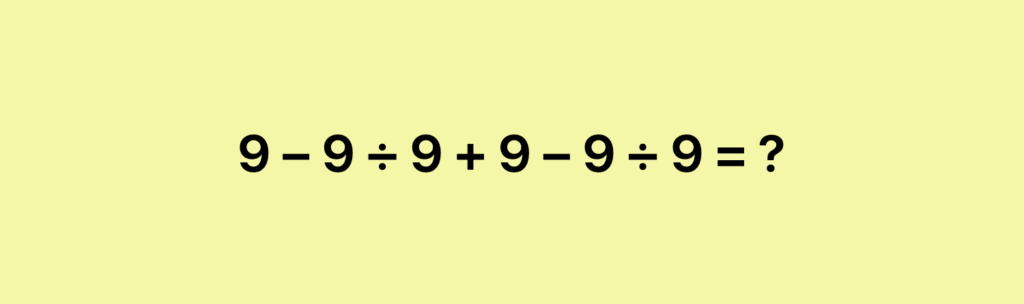
Still feeling confident right there? People online are coming up with a barrage of different answers. It’s gotten to a point where they can’t decide what the correct answer is, but it’s actually a simple PEMDAS operation and the final answer is set in stone.
However, the more contradicting the answers are, the more viral a question will go. Have you solved it yet?
If your answer is zero, four, seven, or a decimal fraction, you might want to take another go at it before taking a peek at the solution. Think of this as a brain teaser, and while they may be a bit pesky sometimes, they are certainly interesting and great for improving your memory and processing abilities.
While you’re at it, let’s talk a bit about how the Order of Operation which is known as PEMDAS (British version – BEDMAS) came to be. It’s not all Euclid and Pythagoras. Our helpful Parentheses/Brackets Exponent Multiplication Division Addition and Subtraction had to have come from somewhere right?
While the actual origin and inventors have remained unknown for decades, historians say that the Order of Operation was created out of a desperate need for simplified solutions. As early as the 6th Century BC, people needed math to keep count of their animals, crops, and carry out trades. Many communities invented their own systems of counting. For instance, the Babylonians worked with a system based on 60, while most others took their measurements in 10s.
Of courses, the only thing they could initially do was to add and subtract. They could lump two groups of sheep together to make one whole group, or remove a few tubers from a heap of crops to have less. However, these two operations were simply insufficient.
They went ahead to invent a method for adding the same repeated number at a faster rate, and multiplication was born. There had to be a way to undo this operation and hence came division.
For centuries, the only thing people did as math was adding, subtracting, multiplying, and dividing.
Over time, there was a need for faster multiplication, and people came up with exponents. How to undo this? Take the root of the operation. They also came up with groupings for clarity such as parentheses and fractional terms.
PEMDAS became a convention when people needed a generally approved method of solving expressions containing multiple operations. It seemed wrong to do whatever a person thought was right when they have to solve something like (3 + 2) x 3 – 4 ÷ 8… There had to be a standard way of getting through it, and that’s how PEMDAS was born. You solve the expression inside grouping symbols such as brackets first, then do exponents and roots, then multiply and divide, and finally add and subtract.
However, addition and subtraction can be interchanged or solved from left to right without any particular order.
The solution to the above question
9 – (9 ÷ 9) + 9 – (9 ÷ 9) = ?
9 – (1) + 9 – (1) = ?
Then we move on to add and subtract from left to right as follows:
9 – 1 + 9 – 1 = ?
8 + 9 – 1 = ?
17 – 1 = 16
The final answer is 16.
Was this what you got? If so, then congratulations! Good job. If you didn’t get the answer correctly, that’s okay. At least you’ve learned something new. Win-win!
Did you get it? Try these next: